A time calculator is an easy and user-friendly calculator that helps us find the time consumed where distance and speed are known.
The relationship between time, distance, and speed is a fundamental concept that plays a crucial role in various fields.
The basic formula that represents the relationship between the three quantities is shown below.
It is also represented by symbols as t=ds, for calculating time when we know about the distance traveled and the speed at which it occurred.
When dealing with complex values or multiple calculations, calculators provide a swift and efficient solution to this problem.This calculator helps in obtaining accurate time calculations with minimal effort. Time calculator can be used in real life applications used in real life scenarios like planning travel routes, duration of a journey and optimizing transportation.
How to use Time Calculator?
We can use this calculator to find the time in two different units of measurement.
- Kilometers per hour
- Miles per second
According to the units of distance and speed, time will be displayed either in seconds or hours.
Kilometers per hour
Step 1: Select the radio button “Kilometers per hour”.
Step 2: In the corresponding text boxes, enter the distance in kilometers and speed in kilometers per hour.
Step 3: Click on CALCULATE and the value of time in hours will be displayed on the screen.
Step 4: To view the thorough calculation, check the Solution box.
Step 5: Click on RESET to start the process again.
Miles per second
Step 1: : Select the radio button “Miles per second”.
Step 2: In the corresponding text boxes, enter the distance in miles and speed in Miles per second.
Step 3: Click on CALCULATE and the value of time in seconds will be displayed on the screen.
Step 4: To view the thorough calculation, check the Solution box.
Step 5: Click on RESET to start the process again.
How to find Time?
Time is equal to the ratio of the distance covered to the speed.
-
Time=\frac{\text{Distance}}{\text{Speed}}
- Distance is the distance traveled.
- Speed is the rate at which the distance is covered.
For example: A car covered a distance of 100 kilometers at a speed of 50 km/h. How long has it traveled?
Distance = 100 kilometers and Speed = 50 km/h.
Time=\frac{\text{Distance}}{\text{Speed}}=\frac{100}{50}=2 hours
So, the car traveled for 2 hours.
Solved Examples
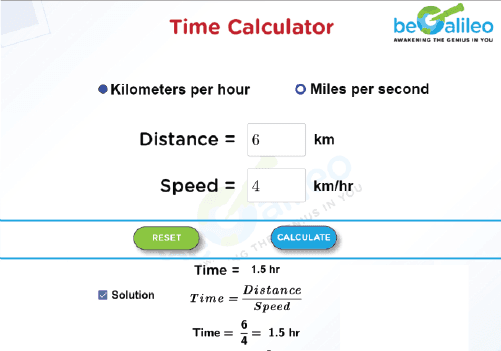
1. Emily is jogging in the park, covering a distance of 6 km. If her speed is 4 km per hour, how long will she spend jogging? f(n)=3^2 for n = 1, 2, 3, 4, 5.
Solution :
Given,Distance = 6 km and Speed = 4 km/hr
Time=\frac{\text{Distance}}{\text{Speed}}
Time=\frac{\text{6}}{\text{4}}=1.5 hours.
Hence, Emily spent 1.5 hours jogging.
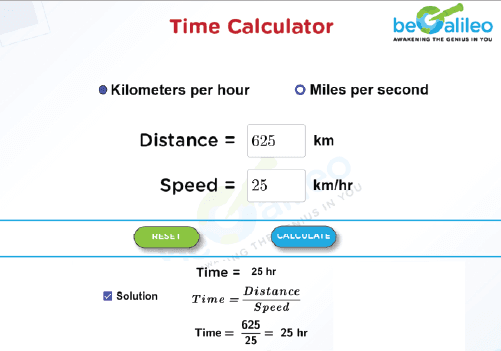
2. How long would it take a person to ride a bike at a speed of 25 km/hr for 625 kilometers?
Solution :
Given, Speed = 25 km/hr and Distance = 625 km
Time=\frac{\text{Distance}}{\text{Speed}}
Time=\frac{\text{625}}{\text{25}}=25 hr.
As a result, the person spent 25 hours.
Frequently Asked Questions on Time Calculator.
1.What time formula do time calculators use?
The formula used is typically Time = Distancespeed , where T is time, Distance is the distance traveled, and Speed is the speed at which the distance is covered.
2.When using a time calculator, which units should I choose for distance and speed?
You should always use the same units. For example, speed should be expressed in kilometers per hour if distance is entered in kilometers.
3.How important is time in physics as a scalar quantity?
Time has simply magnitude and no direction because it is a scalar quantity. When dealing with vector values like displacement in physics equations, time is frequently handled independently.
